4751. Diagonals

In the n × n square table, find the sum of the numbers located on the main and secondary diagonals.

Input. The first line contains an integer n (1 ≤ n ≤ 500). The following n × n matrix consists of integers, each with an absolute value not exceeding 10⁵.

Output. Print the sum of the numbers on the main and secondary diagonals of the matrix.

Sample input	Sample output
4 1 2 3 4 5 6 7 8 3 2 5 4 8 7 9 3	15 21

SOLUTION

2-dim array

Algorithm analysis

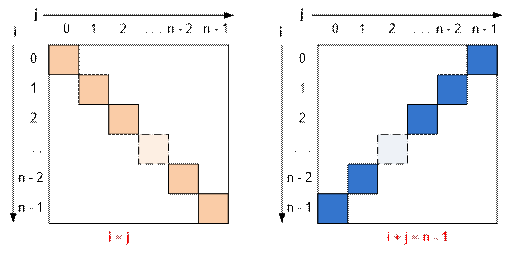
Let m be a given two-dimensional array with zero-based indexing. The element m[i][j] belongs to:

· the main diagonal if i = j;

· the secondary diagonal if i + j = n – 1;

Example

Let’s consider the given example.

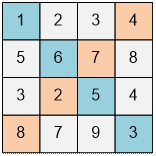
The sum of the integers on the main diagonal is 1 + 6 + 5 + 3 = 15.

The sum of the integers on the secondary diagonal is 8 + 2 + 7 + 4 = 21.

Algorithm implementation

Read the input data. The matrix elements are processed on the fly, without creating a two-dimensional array beforehand.

scanf("%d",&n);

a = b = 0;

for(i = 0; i < n; i++)

for(j = 0; j < n; j++)

{

scanf("%d",&val);

In the variable a, compute the sum of the elements on the main diagonal.

In the variable b, compute the sum of the elements on the secondary diagonal.

if (i == j) a += val;

if (i + j == n - 1) b += val;

}

Print the answer.

printf("%d %d\n",a,b);

Algorithm implementation – two-dimensional array

Declare a two-dimensional array.

int m[510][510];

Read the input data.

scanf("%d", &n);

for (i = 0; i < n; i++)

for (j = 0; j < n; j++)

scanf("%d", &m[i][j]);

In the variable a, compute the sum of the elements on the main diagonal.

In the variable b, compute the sum of the elements on the secondary diagonal.

a = b = 0;

Iterate through all the elements of the two-dimensional array.

for (i = 0; i < n; i++)

for (j = 0; j < n; j++)

{

The element m[i][j] is on the main diagonal if i = j;

if (i == j) a += m[i][j];

The element m[i][j] is on the secondary diagonal if i + j = n – 1;

if (i + j == n - 1) b += m[i][j];

}

Print the answer.

printf("%d %d\n", a, b);

Java implementation

import java.util.*;

public class Main

{

public static void main(String[] args)

{

Scanner con = new Scanner(System.in);

int n = con.nextInt();

int m[][] = new int[n][n];

for(int i = 0; i < n; i++)

for(int j = 0; j < n; j++)

m[i][j] = con.nextInt();

int a = 0, b = 0;

for(int i = 0; i < n; i++)

for(int j = 0; j < n; j++)

{

if (i == j) a += m[i][j];

if (i + j == n - 1) b += m[i][j];

}

System.out.println(a + " " + b);

con.close();

}

}

Java implementation – on the fly

import java.util.*;

public class Main

{

public static void main(String[] args)

{

Scanner con = new Scanner(System.in);

int n = con.nextInt();

int a = 0, b = 0;

for(int i = 0; i < n; i++)

for(int j = 0; j < n; j++)

{

int val = con.nextInt();

if (i == j) a += val;

if (i + j == n - 1) b += val;

}

System.out.println(a + " " + b);

con.close();

}

}

Python implementation

Read the input data.

n = int(input())

m = [list(map(int, input().split())) for _ in range(n)]

In the variable a, compute the sum of the elements on the main diagonal.

In the variable b, compute the sum of the elements on the secondary diagonal.

a = b = 0

Iterate through all the elements of the two-dimensional array.

for i in range(n):

for j in range(n):

The element m[i][j] is on the main diagonal if i = j;

if i == j: a += m[i][j]

The element m[i][j] is on the secondary diagonal if i + j = n – 1;

if i + j == n - 1: b += m[i][j]

Print the answer.

print(a, b)

Python implementation – list comprehension

Read the input data.

n = int(input())

m = [list(map(int, input().split())) for _ in range(n)]

In the variable a, compute the sum of the elements on the main diagonal.

In the variable b, compute the sum of the elements on the secondary diagonal.

a = sum(m[i][i] for i in range(n))

b = sum(m[i][n - 1 - i] for i in range(n))

Print the answer.

print(a, b)